
The amount of compost needed for a planter depends both on planter size, its material and the plant you wish to grow. Compost is important as it will improve your soil’s structure, increasing its available water capacity (AWC), which is especially important for planters. Calculating a planter’s volume (measure in litres) is relatively easy, but it is first important to work out the compost/garden soil ratio required for a particular plant.
Compost/Garden Soil Ratio
Now why do I want to mix compost with garden soil? Firstly, garden soil is incredibly complex with numerous soil organisms that help boost your plant’s health. These organisms will help improve the structure of your soil and break down organic matter into mineral nutrients, available for uptake by plants. However, there is the possibility of inducing pests and diseases, so we recommend avoiding soils that you have previously planted. Secondly, garden soil will help improve drainage, ensuring your planter does not become waterlogged. Lastly, using garden soil will save you money and lower your environmental footprint.
While compost does add nutrients to the potting mix, its major advantage is improving the water-holding capacity of the soil, which occurs through two mechanisms. Firstly, compost contains carbon, as well as other nutrients, that provide food for soil organisms. These organisms function to increase a soil’s porosity – the percentage of soil that is pore space or voids. Secondly, compost improves soil structure by gluing tiny particles of rock (sand, silt, or clay) together into peds (aggregates), which is the basis of all good soils. These peds have adequate pores to allow entry of air and water, both which are essential to plant health. The increased porosity has its origin in the fact compost is significantly lighter than conventional soils.
An ideal soil has a porosity of about 50%, equally divided between micro and macropores, which provides a good mix of drainage and retention. When it rains both macro and micropores become filled with water. Larger pores are the first to drain with light sandy soils taking about a day and heavy clay soils about three. Micropores remain filled and are unaffected by gravitational flow, the water held by electrostatic attraction. The smaller the pore, the more tightly the water is held. Macropores drain too quickly to be of much use to plants, providing little water, but allowing flows of oxygen to plants’ roots. Micropores retain water, available for use by plants. Hence, macro and micropores complement each other, allowing air and water to reach plants’ roots.
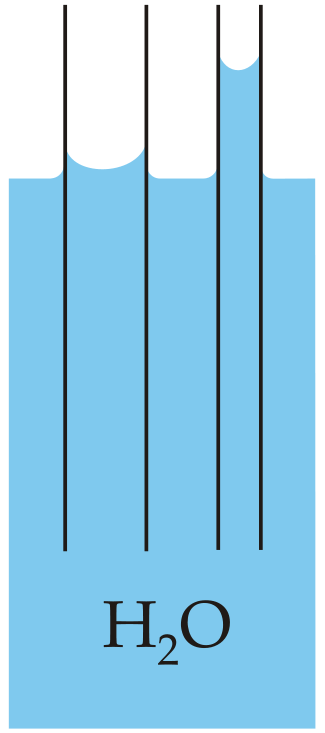
Fascinatingly, small pores act to draw groundwater up through the soil, providing a source of water in the absence of rain. This phenomenon occurs due to the forces of cohesion (propensity of water molecules to stay together) and adhesion (propensity of water molecules to stick to other surfaces). When the force of adhesion is greater than that of cohesion the water rises, with the water near the edge of pore curving upwards. Capillary action can be easily demonstrated by dipping a paper towel in water and watching water climb the towel.
Soil textures – clay, sand, silt and loam – each have different drainage profiles, originating from the size of the particles. Clay particles are the smallest, sand the largest and silt in between. The larger the average particle, the faster the soil drains. Loam is comprised of about 40% sand, 40% silt and 20% clay and is considered the best texture, having the optimal balance of micro and macropores. Clay lacks larger pores, providing poor aeration and drainage, and possesses minute micropores too small for plants to utilise, reducing the soil’s available water capacity. Sand, on the other hand, drains too quickly, predominantly composed of large pores.
Compost increases the number of micro and macropores in the soil, greatly improving a soil’s available water capacity, and should be added to all soil textures including loam. B. D. Hudson’s 1994 paper demonstrated that for every texture as organic matter was increased by 1-3%, the available water capacity doubled. A 2000 study by A. Maynard found that the amount of water in a plow layer (8 inches) increased from 1.3 to 1.9 inches in soil amended with compost, providing a two week supply of water for vegetables, significantly reducing water stress.
An increase in the available water capacity is especially beneficial for potted plants that receive significantly less rainfall due to their container’s small surface area. We recommend the potting mix contain 20-50% compost with higher blends if your soil is clay, your plant thirsty, or the planter’s material porous as with terracotta. Compost will not provide all the nutrients needed, so we recommend the application of organic fertiliser. Mulching is also useful and will help improve water retention and soil structure.
Calculating Volume
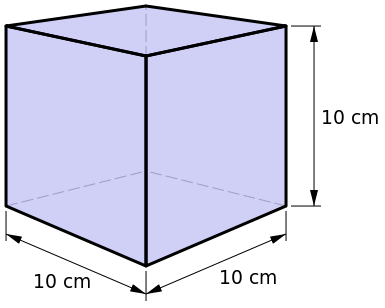
Volume is the sum of 3 measurements (length, width and depth) multiplied together and is expressed in cubic units (cm³, m³). Cubic units correspond directly to litres with 1 litre equal to 1000cm³.
Most planter retailers will give you the dimensions of a planter in cms that can be used to calculate volume. In not, you can use a tape measure. Compost is sold either in litres (l) or cubic meters (m³).
Note: most planter dimensions provided online will be the outer rather than inner dimensions, so you’ll need less compost, depending on the thickness of its sides.
Note 2: planters come in a huge range of shapes. Hypothetically you can calculate the volume of any shape (done by dividing shapes into smaller ones), but we recommend you simply approximate the shape.
Once you have calculated your planter’s litres, simply times it by 0.2-0.5, depending on how much compost you wish to add, to arrive at the quantity you need to buy.
Cubes and Rectangles
Calculating volume for cubes and rectangles is very easy. Simply multiple width, depth and height and then divide by 1000.
Hence, a 100cm³ planter would have a volume of 1000 litres. (100 x 100 x 100 / 1000.) A 140 x 30 x 30 rectangle would have a volume of 126 litres. (140 x 30 x 30 / 1000.)
Cylinders
Calculating the volume of a cylinder requires multiplying height by radius squared by pi which is written as V =πr²h. You then need to divide by a 1000 to get volume in litres. Hence a planter with 30cm diameter and 30cm in height would have a volume of 21 litres. (3.142 x 15² x 30 / 1000.)
Bowls
To calculate the volume of a bowl, you have to calculate the volume of a sphere and divide by 2. Calculating the volume of a sphere requires multiplying 4 divided by 3 times pi times radius cubed, which is written as 4/3πr³. You then need to divide by a 1000 to get volume in litres. Hence a bowl with a 15cm radius would have a volume of 7 litres. (((4/3 x 3.142 x 15³) / 1000) / 2.)
If you would like to know more about soil science please read our guide: Everything you need to know about soil.
If you are interested in pots, Primrose has the biggest range online with over 2000 planters. We also sell compost starting at £5.99.
–
Jorge works in the Primrose marketing team. He is an avid reader, although struggles to stick to one topic!
His ideal afternoon would involve a long walk, before settling down for scones.
Jorge is a journeyman gardener with experience in growing crops.